In collaboration with Y. Brihaye (Mons U)
Study of the Horndeski-Reissner-Nordstrom black hole (HRNBH) solutions. The electric and magnetic types of these BHs differ from each other, since there is no duality symmetry between electric and magnetic fields in the vector-tensor Horndeski gravity. The magnetic black hole solutions are especially simple and can be written explicitly in terms of hypergeometric functions. It is natural to expect that these black holes undergo spontaneous scalarization and it is indeed the case, if we add a massless real scalar field which is coupled to the vector-tensor Horndeski term. The single coupling constant of the Horndeski term is promoted now to a scalar field-dependent coupling function. This system contains (ordinary) Reissner-Nordstrom black holes with a vanishing scalar field, but on a certain “bifurcation surface” in parameter space these solutions become unstable and scalarized HRNBHs appear. The “double-faced” solutions of dyonic scalarized BHs exist in a certain domain of parameter space which is bounded by a critical surface on which the nature of the limiting solutions is determined by the proximity to the lines of vanishing electric or magnetic charges.
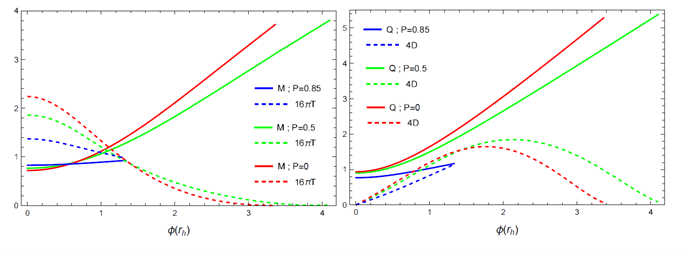
The dependence on the horizon value of the scalar field of the: mass (M), electric charge (Q), scalar charge (D) and Hawking temperature (T) of scalarized Horndeski Black holes with magnetic charges P=0, 0.5, 0.85. Left: mass and temperature, Right: electric and scalar charges.
References:
Y. Brihaye and Y. Verbin, Scalarized compact objects in the vector-tensor Horndeski gravity, Phys. Rev. D102 124021 (2020).
Y. Verbin, Magnetic black holes in the vector-tensor Horndeski theory, arXiv: 2011.02515.
Y. Brihaye and Y. Verbin, Scalarized dyonic black holes in the vector-tensor Horndeski gravity, Phys. Rev. D104, 024047 (2021).